
- #TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES HOW TO#
- #TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES FULL#
- #TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES SERIES#
Calculating Weighted Average in Excel – SUMPRODUCT Function
#TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES HOW TO#
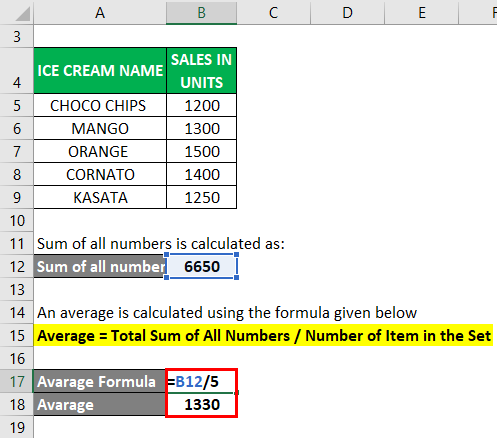
In this tutorial, you’ll learn how to calculate the weighted average in Excel: Calculating Weighted Average in Excel – SUM Function.Example 3 – When the Weights Need to be Calculated.Example 2 – When Weights Don’t Add Up to 100%.Example 1 – When the Weights Add Up to 100%.Calculating Weighted Average in Excel – SUMPRODUCT Function.Many food and energy prices are collected for the off-cycle months, and the unpublished off-cycle indexes are carried forward from the previous month and used in the annual average.
#TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES SERIES#
In addition, users should note that, for an index series that is published every other month, such as those for many metropolitan areas, the annual average is based on 12 months of data. city average series for all items QuantityĪnnual average index value, December 2022Īnnual average index value, December 2021 Table 4, annual average percent change in CPI-U U.S. Users should take care to examine the data with which the CPI is being compared to determine whether the annual average or 12- month percent change is more appropriate for their purposes. city average series for all items is presented in table 4 as an example. The annual average percent change between 20 for the CPI-U U.S. Sum of index values for 2021, divided by 12Ī December-to-December percent change is unlikely to be the same as the change in the annual average percent change between the same two years. city average series for all items Quantity Table 3, annual average of the CPI-U U.S. An annual average percent change should not be confused with the over the year percent change, such as the calculation of the December-to-December change mentioned in the previous section.

Annual averages represent an average index for a given year, not a particular month. city average series for all items is shown in table 3.

As an example, the calculation of the annual average for the CPI-U U.S. If the earlier data point uses the 1982-84=100 base, the later data point must also use that base.Īn over-the-year percent change, such as December 2021 to December 2022, is not equal to the sum of the over-the-month changes between those two time periods.Īnnual averages are the sum of the 12 monthly data points (i.e. There are two critical points to remember when calculating percent changes:Īlways use the same reference base period for all calculations. Table 2, 12-month percent change in CPI-U U.S. city average series for all items from December 2021 to December 2022. Table 2 shows an example 12-month change in the CPI-U U.S. Note: A calculation using January and December data would result in an 11-month change, not a 12- month or year-over-year change. To arrive at a percent change over an entire year, the beginning and ending periods of a CPI series must always be the same month, such as December 2021 and December 2022. Ratio of difference to earlier index value Table 1, 1-month percent change in CPI-U U.S.
#TECHNICAL FORMULA FOR AVERAGE OF PERCENTAGES FULL#
You can find full historical data for this series in our online database. city average series for all items, not seasonally adjusted. Table 1 shows an example of a CPI one-month change between November 2021 and December 2021 using the CPI-U U.S. To find the percent change, you first subtract the earlier index value from the later one, then divide that difference by the earlier index value, and finally multiply the result by 100. Movements of an index from one month to another are usually expressed as percent changes rather than as changes in the index value, because index value changes are affected by the level of the index in relation to its base period, while percent changes are not. Calculating percent changes One-month percent changes


 0 kommentar(er)
0 kommentar(er)
